In early 2011, a pair of theoretical computer scientists at MIT proposed an optical experiment that would harness the weird laws of quantum mechanics to perform a computation impossible on conventional computers. Commenting at the time, a quantum-computing researcher at Imperial College London said that the experiment “has the potential to take us past what I would like to call the ‘quantum singularity,’ where we do the first thing quantumly that we can’t do on a classical computer.”
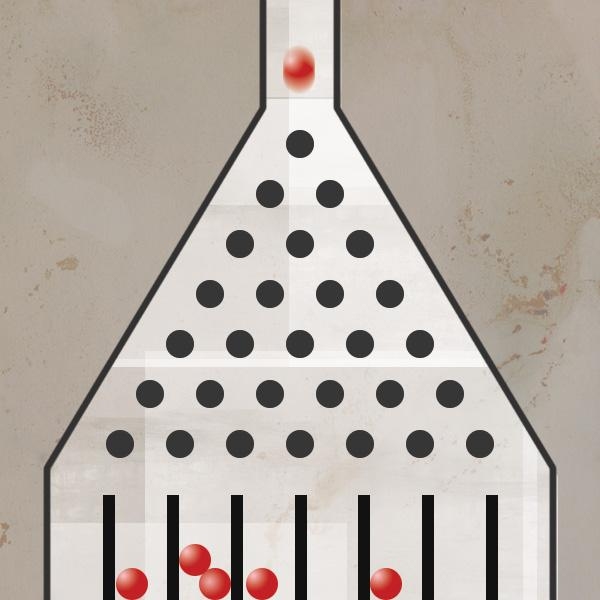
The experiment involves generating individual photons — particles of light — and synchronizing their passage through a maze of optical components so that they reach a battery of photon detectors at the same time. The MIT researchers — Scott Aaronson, an associate professor of electrical engineering and computer science, and his student, Alex Arkhipov — believed that, difficult as their experiment may be to perform, it could prove easier than building a fully functional quantum computer.
In December, four different groups of experimental physicists, centered at the University of Queensland, the University of Vienna, the University of Oxford and Polytechnic University of Milan, reported the completion of rudimentary versions of Aaronson and Arkhipov’s experiment. Papers by two of the groups appeared back to back in the journal Science; the other two papers are as-yet unpublished.
All four papers, however, appeared on arXiv, an online compendium of research papers, within a span of three days. Aaronson is a co-author on the paper from Queensland, as is Justin Dove, a graduate student in the Department of Electrical Engineering and Computer Science and a member of MIT’s Optical and Quantum Communications Group.
Changing channels
The original formulation of Aaronson and Arkhipov’s experiment proposed a network of beam splitters, optical devices that are ordinarily used to split an optical signal in half and route it down separate fibers. In practice, most of the groups to post papers on arXiv — those other than the Queensland group — built their networks on individual chips, using channels known as waveguides to route the photons. Where two waveguides come close enough together, a photon can spontaneously leap from one to the other, mimicking the behavior caused by a beam splitter.
Performing a calculation impossible on a conventional computer would require a network of hundreds of beam splitters, with dozens of channels leading both in and out. A few dozen photons would be fired into the network over a random subset of the channels; photodetectors would record where they come out. That process would have to be repeated thousands of times.
The groups posting papers on arXiv used networks of 10 or so beam splitters, with four or five channels leading in, and three or four photons. So their work constitutes a proof of principle — not yet the “quantum singularity.”
The computation that Aaronson and Arkhipov’s experiment performs is obscure and not very useful: Technically, it samples from a probability distribution defined by permanents of large matrices. There are, however, proposals to use optical signals to do general-purpose quantum computing, most prominently a scheme known as KLM, after its creators, Emanuel Knill, Raymond Laflamme and Gerard Milburn.
According to Dove, some in the quantum-computing community have suggested that Aaronson and Arkhipov’s experiment may be difficult enough to perform with the requisite number of photons that researchers would be better off trying to build full-fledged KLM systems.
But, Dove says, “One of the ways that Scott and I like to pitch this idea is as an intermediate step that we need to do KLM.” Building a KLM optical quantum computer would entail building everything necessary to perform the Aaronson-Arkhipov experiment — plus a bunch of other, perhaps even more challenging, technologies.
“You can think of Scott and Alex’s result as saying, ‘Look, one of the steps to performing KLM is interesting in its own right,’” Dove says. “So I think it’s inevitable that we’re going to do these experiments, whether people label them that way or not.”
The experiment involves generating individual photons — particles of light — and synchronizing their passage through a maze of optical components so that they reach a battery of photon detectors at the same time. The MIT researchers — Scott Aaronson, an associate professor of electrical engineering and computer science, and his student, Alex Arkhipov — believed that, difficult as their experiment may be to perform, it could prove easier than building a fully functional quantum computer.
In December, four different groups of experimental physicists, centered at the University of Queensland, the University of Vienna, the University of Oxford and Polytechnic University of Milan, reported the completion of rudimentary versions of Aaronson and Arkhipov’s experiment. Papers by two of the groups appeared back to back in the journal Science; the other two papers are as-yet unpublished.
All four papers, however, appeared on arXiv, an online compendium of research papers, within a span of three days. Aaronson is a co-author on the paper from Queensland, as is Justin Dove, a graduate student in the Department of Electrical Engineering and Computer Science and a member of MIT’s Optical and Quantum Communications Group.
Changing channels
The original formulation of Aaronson and Arkhipov’s experiment proposed a network of beam splitters, optical devices that are ordinarily used to split an optical signal in half and route it down separate fibers. In practice, most of the groups to post papers on arXiv — those other than the Queensland group — built their networks on individual chips, using channels known as waveguides to route the photons. Where two waveguides come close enough together, a photon can spontaneously leap from one to the other, mimicking the behavior caused by a beam splitter.
Performing a calculation impossible on a conventional computer would require a network of hundreds of beam splitters, with dozens of channels leading both in and out. A few dozen photons would be fired into the network over a random subset of the channels; photodetectors would record where they come out. That process would have to be repeated thousands of times.
The groups posting papers on arXiv used networks of 10 or so beam splitters, with four or five channels leading in, and three or four photons. So their work constitutes a proof of principle — not yet the “quantum singularity.”
The computation that Aaronson and Arkhipov’s experiment performs is obscure and not very useful: Technically, it samples from a probability distribution defined by permanents of large matrices. There are, however, proposals to use optical signals to do general-purpose quantum computing, most prominently a scheme known as KLM, after its creators, Emanuel Knill, Raymond Laflamme and Gerard Milburn.
According to Dove, some in the quantum-computing community have suggested that Aaronson and Arkhipov’s experiment may be difficult enough to perform with the requisite number of photons that researchers would be better off trying to build full-fledged KLM systems.
But, Dove says, “One of the ways that Scott and I like to pitch this idea is as an intermediate step that we need to do KLM.” Building a KLM optical quantum computer would entail building everything necessary to perform the Aaronson-Arkhipov experiment — plus a bunch of other, perhaps even more challenging, technologies.
“You can think of Scott and Alex’s result as saying, ‘Look, one of the steps to performing KLM is interesting in its own right,’” Dove says. “So I think it’s inevitable that we’re going to do these experiments, whether people label them that way or not.”