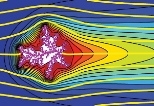
Imagine a rock in a stream. Water is flowing over the rock, and sticky little particles diffusing through the flow adhere to the rock as they go by. Initially only one or two particles stick to one point on the rock. Then, as more accumulate, the particles start to form long tendrils resembling octopus arms, and later, tree branches that taper into tiny twigs.
The result is this remarkable fractal image created when Martin Z. Bazant, associate professor of applied mathematics, and his group applied a new mathematical twist to a model called diffusion-limited aggregation (DLA). As the conglomerate of particles grows, the effect of the flow becomes more pronounced. The tips on the front side facing the particle-laden flow (blue) get more action than the tips at the back. At the back, you can see the effect of the flow as the region depleted of particles (red) is swept downstream.
Scientists use models like DLA to explain pattern formation. In narrow faults inside rocks, you might find a two-dimensional dendritic crystallization pattern like this one, composed of deposited materials. If you knew about flow, you could work backwards and infer the flow profile on a geological time scale, which cannot be directly measured. The new models also may shed light on other fractal patterns in electrodeposits or semiconductor surfaces, which involve electric fields rather than fluid flow.
A version of this article appeared in MIT Tech Talk on February 4, 2004.